Structure of the electron
Electromagnetic
Model of the Electron
Dr. Christoph Caesar
D-81677 München, Buschingstrasse
30, Germany, physics(a)ccaesar.com
19.01.2009, updated
1.4.2023
To date, no detailed accepted theory
or model of the electron itself is established. What is
known is a variety of properties, each standing alone in
a table of elementary constants.
A physically realistic model of the
electron revealing properties like "spin" and
"charge" e- will be of substantial
interest to the world of quantum physics. Reducing the number
of these constants is of the greatest interest. In this
work, the electron is described as circulating electromagnetic
wave with an internal twist like a Moebius band or Moebius
ribbon. The positive half wave stays on the inside and partly
compensates itself in a way that the negative field of the
wave always is on the outside. The ratio of the field energy
responsible for the charge to the total particle rest energy
is the dimensionless figure 1/137, which is identical in
value and formula to the fine structure constant. This formula
has been derived purely empirically and is supposed to give
the elementary charge in "natural units" - whatever
that means. In this work, it will be shown - among some
other things - that a physical, deterministic and logical
model gives a derivation of this mysterious fine structure
constant from .
The electron model as rotating and
inverted photon does not contradict current models and offers
a great simplification by using a strictly deterministic
mind set.
I. INTRODUCTION
Various disciplines of physics have been concerned with
the investigation of structure and reactions of leptons
and hadrons for decades. Higher and higher energies were
used in order to examine matter with increasing resolution.
Despite the tremendous intellectual and financial effort,
however, a realistic model or a physical description of
the fundamental quantum characteristics of a quark or electron,
as spin or charge, has not been found yet. There is a strong
motivation to find a model of the electron since its discovery,
from the begin of elementary particle physics to basic lectures
of physics and chemistry at today's universities. R. Gross[1]
describes several approaches to find reasonable properties
of the electron based on the classical electron radius and
states that they "lead to no reasonable result" applying
laws of classical physics. Even if physics and chemistry
can describe exactly the probability distribution of the
electrons around the nucleus it is still open of what they
describe the probability of occurrence. At present, the
electron is a "black box" with abstract quantum properties.
The box has an unknown size or diameter, it has a property
called "Spin" and it exhibits two variations of the spin
(+1/2 h-bar and -1/2 h-bar), each time it enters an asymmetric
magnetic field. It shows an external electrical field which
is not correlated with its mass or spin and is defined as
the elementary constant e-.
Several approaches for a quantum reality of the electron
have been published, e.g. a model with mass less particles
circulating each other with the speed of light[2]
or a model which regards the electron as a solid charged
shell rotating with the speed of light[3].
These models, however, cannot predict the elementary charge
or the correct spin nor could the existence of the mass
less particles be proven. A solid shell or mass, however,
has never been observed to move with c, either.
A model of the electron should further be able to reduce
the number of the elementary constants (now approx. 20)[4].
If merely the electron mass, spin or elementary charge could
be correlated with each other this would be regarded as
substantial progress according to some authors[5],[6].
II. THE ELECTRON
What is the electron? What is a rotating charge cloud with
a negative electrical field, which behaves like a wave in
interference experiments and can expel photons from some
substances? A microscopic pebble with charge (classical
assumption for the search for a solid particle with finite
diameter)? An abstract quantum wave of statistical nature
as placeholder wave? What is a rotating charge cloud with
a negative electrical field, which behaves like a wave?
An electromagnetic wave circulating with the speed of light
"c"? If the negative field of the electron were formed as
part of an electromagnetic wave, in which the negative part
of the electrical field always is on the "exterior" and
where the positive half wave remains on the inside somehow
hidden, such a structure or particle wave electron could
be imagined, i.e. a rotary or "wound up" photon. The
energy of the electromagnetic field entirely forms the
mass equivalent of the electron in this model.
How does the field of an electromagnetic sine wave look
like? The electric field is defined as the direction in
which a test charge would move; the intensity of the field
per definition corresponds to the acceleration the test
charge is subjected to. Along the path of the wave, the
field strength corresponds to the classical sine wave as
given in fig. 1.
Fig. 1: Electrical field strength E of an
electromagnetic sine wave. Right side: Vector field of E
in the x-y plane
(z-axis out of plane): the vectors give
the direction and magnitude of the acceleration of test
charges
If looked at in space (Fig.
1 right side), the field has an underside, and the field
strength is defined as the length of the vector. The positive
test charge above the x - axis (x-z - plane) is repelled
by the field of the positive half wave into the direction
of the arrows, i.e. the field of the positive half wave
acts like a positive charge "above" the plane. The same
test charge, however, is drawn upwards, if placed below
the path of the wave in the x-z plane. This, per definition,
is the action of a field that attracts the positive test
charge, a negative field. The following negative half wave
now attracts the positive test charge above, but acts as
positive field geometrically below the plane.
Fig. 2: Path of the
rotating electromagnetic wave forming the electron in shape
of a Moebius ribbon
A possible construct for a particle that is "always" negatively
charged (on the outside) and has the positive part somehow
hidden on the inside can be imagined, if the wave turns
upside down after one half phase. Fig. 2 schematically shows
a Moebius ribbon as path of such a circulating electromagnetic
wave with one internal torsion per revolution. The ribbon
could also be a segment of a spherical wave in reality,
but the internal twist of the zero transition is harder
to visualize in a sphere. Therefore the model of the ribbon
is kept in the further. The field intensity has the maximum
of one half sine on the right and a zero transition. It
shows the internal torsion of the Moebius ribbon. The field
is perpendicular to the surface; the normal vector would
give the direction, in which an assumed test charge would
move.
Although there are no smaller test charges than the electron
itself, this is a permissible thought experiment. The internal
torsion of the Moebius ribbon ensures that only the negative
half wave is outside and after zero transition the „lower
surface" of the positive half wave is on the outside, which
is again negative from their effect.
The field of any photon or wave is compensated in the long
range effect over space and time by the field of the next
half wave, so it is electrically neutral. The rotating construct
discussed here should be neutral, too. However, the positive
inside field - in case of the electron - propagates through
the diameter. The field component which is exactly in the
plane is counteracted by the positive field of the next
revolution pointing in the opposite direction. All out-of-plane
positive and negative field effects are assumed to compensate
themselves in larger distances, too. The field strength
in radial direction Er in polar coordinates of
the Moebius ribbon surface (r, Phi and Psi) schematically
is of the type
Equation (1)
which mathematically always is positive. The angles Phi
and Psi can be regarded as identical. The field strength
E decreases with 
,
which is not regarded in the graph below in the first place.
The model matches the relativistic four-component Dirac
spinor (thanks to Oskar Axelsson for this input (personal
communication)).
Fig. 3 shows the vectors in the plane of rotation of the
wave for an assumed starting time t = 0 and for the time,
when the effect of the positive field emitted at t = 0 has
reached the other diameter with c. The wave then has passed
an angle of 114.59 degrees, at which the arc length equals
the diameter. A certain fraction of the external negative
field then cannot be compensated in the long range and forms
the external field of the particle. This fraction will be
assessed mathematically below.
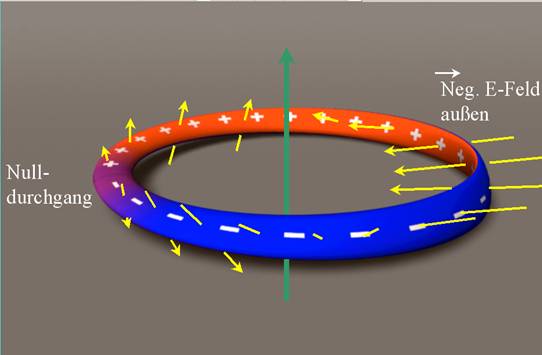
Fig. 3 Rotating vector field in the plane of the ribbon for
time t = 0 . For t = t0 + Pi/2 the positive field compensates
itself partially. (Graph to be completed). The radial component
has a cos2 or sin2 amplitude, which
are always positive, as well.
This rotating photon of the current model is suggested
to be called "c-tron" because of the peripheral speed of
light c.
III. PROPERTIES AND VARIATIONS OF THE C-TRON
The field rotates around its axis - therefore the particle
has a spin. During one revolution of the field along the
path of the Moebius ribbon only the first half of the sine
wave is accomplished. The second half wave is completed
after the internal turn during the second revolution. It
is a very remarkable property of this model that two revolutions
are necessary, in order to accomplish a full cycle or phase.
This is the definition of the spin 1/2! The fact that some
quantum particles need 2 revolutions to perform one full
phase fills pages in physics books. The strange phenomenon
is described with the analogy as if the earth needed 720
instead of 360 degrees to have turned completely. This abstract
quantum feature clearly is met by this model.
The ribbon loop can exist in two variations: with an outward
torsion with respect to the direction of revolution and
with an inward torsion seen in the same direction. The same
can be imagined for the positron. If the field rotation
is counter clockwise in Fig. 2, the torsion is shown as
outward. An additional magnetic moment results from the
change of the field orientation at the zero transition.
This additional moment is different, if the torsion goes
inward. In an external magnetic field, this moment will
divert the c-tron either "up" or "down" in the magnetic
field, representing the "spin up" and "spin down" leptons.
The particle further can have the negative field or the
positive field on the outside, thus representing the electron
or the positron (see Figs. 2 and 4). The field of a circulating
wave without internal torsion is negative in one revolution
and positive within the next one, thus altogether neutral
and regarded a candidate for the neutrino.
The model offers photons and leptons in a unified structure:
as linearly propagating photons and as circulating photons
or c-trons. The latter occur with internal torsion as electrons
or as positrons, each with spin up or down, or consequently
as neutrinos with no internal torsion.
The change of the electric field induces a magnetic field
which has its maximum at the zero transition of the electric
field. It can be imagined that a closed loop of the magnetic
field is formed - see fig. 4. The similarity with the well
known picture of the electron as small bar magnet is compelling.
The paths of the magnetic fields form a figure "eight" during
one full phase and the electric field passes the circumference
twice. It is this synchronizing of the radial frequency
of the magnetic field with the tangential frequency of the
electric field that might ensure the stability of the particle.
FIG. 4: Schematic
formation of the magnetic field of the positive c-tron in
three steps with the classical notation of the electron
as tiny magnet (lower right**)
(** The magnetic field still
is wrong in the animation, as the magnetic field lines follow
the edge of the ribbon as envelope of induced vortices by
the Maxwell equations, but basically the magnetic field
lines do pass through the inner side of the ribbon. Thus
the consequences and the notation of the electron as nanoscopic
rod magnet can be kept (to be updated, 17.04.2013).
This aspect is supported by the model of Dr. Qiu-Hong Hu
on the "the nature of the electron" as Hubius
Helix. The Hubius Helix is the "rim" of a moebius
ribbon and perfectly corresponds to the corrected magnetic
field of the model of the electron.
see http://arxiv.org/ftp/physics/papers/0512/0512265.pdf
This model of a circulating wave so far shows all characteristics
of the electron:
IV. RADIUS DETERMINATION FROM SPIN
As a test of this model it is examined whether characteristics
like the spin are associated with the mass of the electron,
if the mass equivalent of the electromagnetic field rotates
with speed of light „c". The classical electron radius is
derived from the capacity of the electron as sphere condenser
with charge e:
Equation (2) after: Mohr & Taylor[7]
The equatorial peripheral speed v of this classical electron
would exceed the speed of light in several approaches, although
it is a postulate of quantum mechanics not to contradict
the classical mechanisms. The classical approach leads to
"no meaningful result" according to the own statements of
modern physics (R. Gross[8]).
The peripheral speed „v" in the current model is assumed
as speed of light „c". This approach is strictly forbidden
according to the standard model, since no „mass" can move
with speed of light. Only „mass less" particles or photons
which nevertheless are provided with an exactly defined
mass/energy equivalent can move with c. If the approach
is wrong, it should therefore lead to an unreasonable result.
The angular momentum L of a mass (e.g. mel)
around an axis and the radius r is defined as: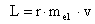 
The mass of the electron mel (= field energy
/c2) rotates with „c" around the radius "r" in
a first approximation. With v = c and with 
the angular momentum L becomes 
.
The electron spin is defined as 
.
Now the radius is the only unknown parameter and can be
calculated:
Equation (3)
which gives
.
This radius corresponds to a measured value for the scattering
of an individual photon at the electron. The quantity 
m
is well known as Compton wavelength and also has been determined
as electron diameter by Giese[9]
and Mills[10]. What,
if the Compton wavelength were more than a historical observation
without further meaning? It should be considered that the
analysis of atomic distances with x - rays is a usual procedure
in physics and e.g. metallurgy. The measured Compton wavelength
according to this model becomes a measurement of the diameter
of the circulating wave of the electron.
The computation of the electron radius from the spin as
rotation of the mass/ energy of the electromagnetic field
with speed of light "c" around the radius "r" leads to a
meaningful result in contrast to classical computations
of the electron radius.
There are plenty of data suggesting that the diameters
of electrons, protons or neutrinos are below a certain size
of e.g. 10-15 m. This would certainly be correct
if looking for the small "stone with charge". It should
be regarded, however, that the diameter will decrease at
increasing energies of the particle/ c-tron. An electron
accelerated to 1 GeV total energy has a diameter of 1.9
* 10-16 m according to eq. 3, which perfectly
fits to the current model and to the observations concerning
measured particle diameters or effective cross sections
at high particle energies.
The radius determined before has to be compatible with
the classical quantum physics observations as the de Broglie
wavelength and the mass energy equivalence. One full phase
of the c-tron of the current model is completed after passing
the circumference "C" of the particle twice, i.e. 
.
With the electron diameter d = 2 r = 3,86 * 10-13
m derived from the spin, the wavelength can be calculated
to 
=
2.425 * 10-12 m and with 
the circulation frequency of the particle in rest to 
.
It is interesting that this rest mass frequency often is
cited in physics books, but its nature rarely is explained
or commented[11].
In a very clear way the natural frequency 
by
de Broglie for an electron in rest gets a realistic meaning
as the true frequency of the c-tron of the energy 
.
In the model, the c-tron wavelength of 
differs from the de Broglie wavelength of an electron of
1 eV (5.92 105 m/s) of ca. 10-9 m.
The de Broglie wavelength of a moving particle, however,
is interpreted as the group velocity of matter waves. It
is assumed that the displacement of the E
- field maximum of the circulating wave for a certain particle
velocity is identical with the de Broglie wavelength.
The fraction 1/X of the field energy of the c-tron that
forms the external charge to the total field energy can
be calculated. The electron is regarded as sphere capacitor
with the stored energy E = 1/2 Q2 /C.
.
With the capacity
,
the charge Q is
Equation (4)
With the values for the de Broglie frequency 
,
the electron radius 
determined above and the factor 1/X = 1/137, the electron
charge is calculated correctly to 1.603 10-19
C. The dimensionless fraction 1/X equals the fine structure
constant
,
whose derivation may have been similar to the above approach.
The figure 1/137 could be coincidence, too. If, nevertheless,
the known formula of 
is inserted into eq. 4, the product inside the root equals
to 
and Q = e. The coupling constant
perfectly confirms the current model.
Above approach is regarded the quantum realistic origin
and meaning of the coupling constant as the ratio of the
electric field energy forming the charge of the electron
to the total field energy 
.
V. C-TRON MODEL AND QUANTUM MECHANICS
The interpretation of the electron as a circulating wave
or "photon" leads from one elementary constant, the spin
of the electron, to the elementary charge using another
well known dimensionless factor, the fine structure or coupling
constant. It gives a realistic meaning to the spin up and
down characteristics and provides an approach for the neutrino.
The model solves the mystery of the application of the
equation 
to solid matter, which led to the wave aspects and the de
Broglie wavelength of matter. At the same time it opens
a door to the equivalence of energy and matter itself, E
= m c2. Electromagnetic energy and matter are
equivalent because they are of identical nature, at least
shown for leptons so far. The application to hadrons or
quarks in a similar approach is assessed elsewhere[12].
The consequences of the model are the following:
o The particle can behave as a wave, as it is a wave. Nevertheless
it has the mass of m=E/c2, which can cause all
effects a solid particle can cause.
o As the field revolves with the speed of light c, a macroscopic
prediction of the results other than with the statistical
methods of quantum mechanics is hardly possible.
o The Zeeman Effect is the effect of the c-tron oscillating
around the nucleus in reality.
o There is a real sense of rotation in c-tron particles.
In collision experiments, a parity violation must be observable
due to superposition of the local field strength - The decay
of W+ or W- particles exactly shows this effect: These can
only decay into leptons with matching helix direction[13].
o The fine structure constant 
is the fraction of uncompensated field energy (charge) to
total field energy. 
should therefore increase, if the proportion of the total
rotating field that is effective for an interaction increases
e.g. for small distances to the rotary electromagnetic field
or for high energies. This is exactly what is found[14].
o The model describes matter as closed loops of electromagnetic
waves, which do not have a point like centre and therefore
are free of singularities. Mathematical problems in dealing
with point-like mass concentrations therefore are eliminated.
o The old question why the electron accelerated on its
path around the nucleus shows no emission of energy is solved:
no charge circles the atom but an electromagnetic wave with
some excess field on the outside.
The model presented is based solely on the definition of
the electric field itself and on classical equations of
physics and early quantum physics. The model offers a great
unification and simplification for the quantum world of
the leptons so far.
VI. EXPERIMENTAL VERIFICATION
Experimental verification to verify or falsify a basic
concept is required for every new theory. Absolutely identical
to proposing new experiments is the requirement that a theoretical
model shall fulfill the results of experiments already performed
- if it offers simplification compared to the assumptions
required by the old theory.
The natural and realistic explanation of the spin 1/2 is
a strong aspect in favor of the current particle model.
The experiments proposed would have been to observe the
spin balance in particle collision experiments and watch
for the law of spin conservation with the postulated spin
½ for the electron.
All formulations and correlations between e.g. charge and
fine structure constant have already been found. The formula
for
had been found earlier - but with what interpretation? Here
the interpretation is given in a simple and realistic way.
An oscillating movement of the electron and the interaction
with the magnetic field of the nucleus - the Zeeman-effect
- already has been discovered. Fine and hyperfine structures
in the spectra of light emitted by atoms had been found
and interpreted in this way. In the c-tron model it can
easily be visualized that each of the small loops or ripples
on the orbit[15]
corresponds to one field rotation with the De Broglie frequency.
The Moebius ribbon has another remarkable property, which
was found experimentally: a set of eight paper ribbons was
produced, which were marked with a polarity - red or black
for positive or negative field, both pointing up and down,
all twisted either to the left or to the right before closing
the loop with paper adhesive in identical manner. Table
1 shows the results of this little experiment.
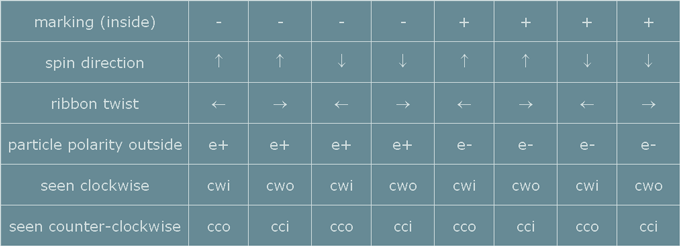
TABLE 1: All possibilities of making
a paper strip Moebius ribbon. "cwi" and "cwo" is seen clockwise
with inward, respectively outward torsion, "cci" and "cco"
are the same seen counter-clockwise.
There are four positive and four
negative combinations. Basically they contain two groups
either being the clockwise inward or clockwise outward type.
Each particle has the property "inward" torsion and "outward"
torsion at the same time. It shows an inward twist if observed
from one side and outward twist if seen from the other side
in space. This means that each beam of electrons will sort
itself into two beams in a Stern - Gerlach experiment. Each
split beam, which is allowed to loose its orientation in
space (having been aligned by the magnetic orientation),
will have - again - both properties of inward torsion and
outward torsion depending on the orientation of the individual
particle as it enters a next quantum separator. This is
exactly what is been found experimentally.
As usually a continued split up of
every filtered beam in two beams is observed, it might be
worth while looking at the results under the aspect of the
current model. The + and - spin directions classically should
be identical in magnitude and energy and differ only in
the sense of rotation. The c-trons might show different
energy levels with the twist inward or outward and stay
that way if filtered once. Any splitting of the beam from
then on would have to be attributed to a randomization of
the orientation between the filters and a sorting in the
next magnetic field depending on how they enter this field.
There are experiments to verify the
model, e.g. the existence of the two basic versions of the
electron with torsion outwards and torsion inwards to give
an additional magnetic moment. It already has been performed
- the Stern Gerlach experiment led to the description of
the properties "spin up" and "spin down".
Experimental verification of the
proposed model can be performed with a Stern - Gerlach type
of experiment, too, where first the clockwise-inward are
sorted from the clockwise outward electrons. Then one beam
can again be sorted in two, if the electrons are allowed
to randomize - e.g. by thermal movement, by interaction
with other magnetic fields or by gaps in the magnetic field
keeping the alignment.
If, however, the electrons are e.g.
cooled and the orientation in the magnetic field constantly
is kept aligned, the filtered beam should not spilt in two,
again. Extremely low energy electrons with low beam densities
should be preferable in this test, no interaction with magnetic
fields of nearby atoms or neighboring electrons can be allowed.
Proposed for verification further
is a three dimensional, time dependent field simulation
to show the statistical - like behavior of the c-tron in
collision experiments. It cannot behave other than the observed
quantum waves; however, interactions are strictly local
and causal.
Second, mathematical integration
over volume and time of the field of the wave circling the
Moebius ribbon (e.g. based on eq. 1) is proposed. It should
prove that 1/137 of the total field-energy and mass equivalent
of the electron of eq. 2 are sufficient to form the field
of the elementary charge, stipulated that E0
is determined from another source.
There is no accepted formulation
for the structure of the electron, rather than effort to
avoid this crucial question by using the abstracted "black
box" with quantum properties. The current formulation of
the electron is a basic concept and is not in contradiction
to accepted theories.
LIST OF FIGURE CAPTIONS
FIG. 1: Electrical field strength E of an electromagnetic
sine wave (left side).
Right side: Vector field of E in the x-y plane (z-axis
out of plane): the vectors give the direction and magnitude
of the acceleration of test charges
(Color online) FIG. 2: Path of the
rotating electromagnetic wave forming the electron in shape
of a Moebius ribbon
FIG. 3: Rotating vector field in
the plane of the ribbon for time t = 0 and for t = t0 +
Pi/2. The radial component has a cos2 amplitude.
(Color Online) FIG. 4: Schematic
formation of the magnetic field of the positive c-tron in
three steps with the classical notation of the electron
as tiny magnet (lower right).
FIG. 5: Comparison of natural twist
of a Moebius ribbon with the classical electron orbit.
REFERENCES
[1]
R. Gross, Das Wasserstoffatom, http://www1.physik.tu-muenchen.de/~kressier/SS02/phy34/4.pdf,
Chapter 4
[2]
A. Giese, Proc. Spring Meeting of the DPG, Dresden,
Germany, (March, 24, 2000), cited after http://www.ag-physics.org
[3]
R. L. Mills, http://www.blacklightpower.com/theory/book.shtml
[4]
F. Grotelüschen, Der Klang der Superstrings, dtv,
München,(1999), p. 30
[5]
O. Höfling, P. Waloschek, Die Welt der kleinsten Teilchen,
8474, rororo, Reinbek bei Hamburg, (1988), p. 455
[6]
F. Grotelüschen, Der Klang der Superstrings, dtv,
München, (1999), p. 30ff
[7]
Mohr & Taylor Physical Constants, (1999) http://pdg.lbl.gov/2000/consrppbook.pdf
[8]
R. Gross, Das Wasserstoffatom, lectures, http://www1.physik.tu-muenchen.de/~kressier/SS02/phy34/4.pdf,
Chapter 4
[9]
A. Giese, (Spring Meeting of the DPG, Dresden, Germany March,
24, 2000), after http://www.ag-physics.org
[10]
R. L. Mills, http://www.blacklightpower.com/theory/book.shtml
[11]
W. H. Westphal, Physik, Springer Verlag Berlin
(1963), p. 620
[12]
C. R. Caesar, Elektromagnetisches Teilchenmodell sowie
Verfahren zu seiner Berechnung und Simulation, (submitted
2003.09.08, published 2005.04.14) German Patent Application
DE 103 41 341 A1
[13]
O. Höfling, P. Waloschek, Die Welt der kleinsten Teilchen,
8474, rororo, Rheinbek b. Hamburg 1984, p. 420
[14]
http://www.didaktik.physik.uni-erlangen.de/grundl_d_tph/lexikondf.html#Feinstrukturkonstante
[15]
R.Gross, Das Wasserstoffatom, http://www1.physik.tu-muenchen.de/~kressier/SS02/phy34/4.pdf,
p. 133
Web Counter by www.webcounter.goweb.de
STRUCTURE
OF THE ELECTRON
,
which can "impact" to other materials and interact like
a particle